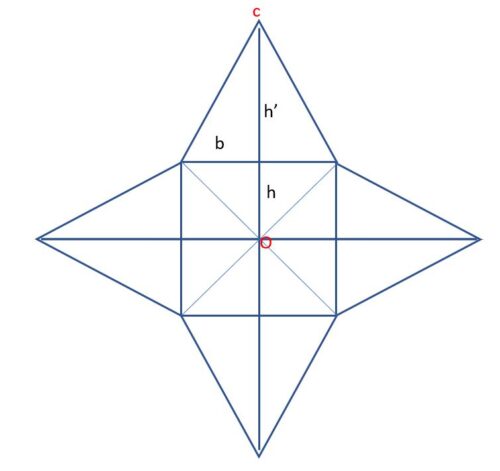
The Area of the perfect star shape is,
\begin{equation}
A =\frac{1}{2} nbD \>\>\>\>(1)
\end{equation}
Where n is a number of vertices, D is a diagonal from original o to vertex c and b is the base of triangles.
Here the perfect start shapes mean that the diagonals in all triangles to original are equal.
Proof:
The area is,
\begin{equation}
A_1 =\frac{1}{2} {bh} +\frac{1}{2} {bh’}
\end{equation}
\begin{equation*}
A_1=\frac{1}{2} b(h+h’)
\end{equation*}
Where, h+h’=D, Thus,
\begin{equation} \label{eq:mak}
A_1 = \frac{1}{2} bD \>\>\>\>(2)
\end{equation}
Then the Area of Star shape is a total of all triangles which is,
\begin{equation}
A=\frac{1}{2} {nbD}
\end{equation}
The area for four vertices is,
\begin{equation} \label{eq:mako}
A=\frac{1}{2} (4 bD)={2bD} \>\>\>\>(3)
\end{equation}
The area for five vertices is,
\begin{equation} \label{eq:makoo}
A=\frac{5}{2} {bD} \>\>\>\>(4)
\end{equation}